NLSM 3D AMR Research

Movie of near critical evolution: (4/17)

Toroid (Doesn't show coarsest grid)

X-Y Plane; Smooth Mesh (1MB MPG)

Y-Z Plane; Smooth Mesh (1MB MPG) 1D Cuts through X and Z axes; (6MB MPG)

Recent Movies: (4/02)

Squished Toroid	X-Y Plane; Smooth Mesh (4MB MPG) X-Y Plane; Wire Mesh (4MB MPG)	Y-Z Plane; Smooth Mesh (4MB MPG) Y-Z Plane; Wire Mesh (4MB MPG)
Two colliding pulses	X-Y Plane; Smooth Mesh (3MB MPG)	X-Y Plane; Wire Mesh (3MB MPG)
Spinning Ellipsoidal Pulse (Spinning about z-axis) Singularity Forming	X-Y Plane; Smooth Mesh (0.5MB MPG)	X-Y Plane; Wire Mesh (0.5MB MPG)

More Recent Results: (11/01)

• Near critical evolution (0.5 MB).
• Zooming in at critical time on above solution (0.3 MB).
• Monopole (0.3 MB) pair collapse in a different model (easily adapted from NLSM code).

Preliminary Results: (8/30/01)

• Movie of near-critical chi (z=0 slice). Any self-similarity demonstrated by the solution is not clear in this movie because it's hard to see the dynamics on the various scales. However, the following demonstrates it more clearly at the expense of cutting out much of the data.
• Movie of near-critical chi. Here, an x=0, y=0, z>=0 cut is shown versus log(r). This is analogous to such plots done with explicitly spherically symmetric adaptive codes. The solution shows the familiar self-similar behavior chi(r/t).
• Movie of chi with bounding boxes on the various grids (z=0 slice).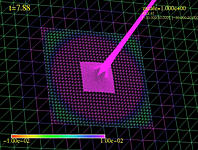
• Movie of the near-critical energy density (z=0 slice).

Additional Notes:

One other thing about the above. In this nlsm model, I use a generalized hedgehog ansatz which requires somewhere that the field goes to zero. I fix the field to be zero at the origin, and that's why you see the zero point at the origin in the above.